305,5013,30592,105763958 ?

What is the next number in this sequence: 305,5013,30592,105763958,__?

200 Fishes in Aquarium Puzzle

There were 200 Fishes in an aquarium, 99% of which were red. How many red fishes must be removed to make the percentage of red fishes 98%?

Guess the Computer Password ?

A man locked his personal computer with a password and wrote some phrases in the hint box. One day his wife tries to login in his absence using the hints which contained following: 4 grapes 1 apple 7 bananas 7 mangoes 2 pineapples 1 orange 8 pomegranates

What is the password?

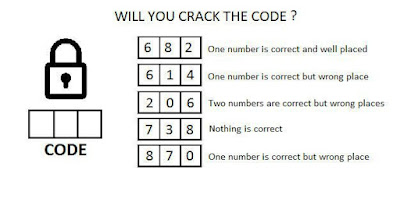
WILL YOU CRACK THE CODE ?

Hint1 - 682: One number is correct and well placed
Hint2 - 614: One number is correct but wrong place
Hint3 - 206: Two numbers are correct but wrong places
Hint4 - 738: Nothing is correct
Hint5 - 870: One number is correct but wrong placed

Can you crack the lock code with the help of the hints given ?

Find the 9 letter word _a_s_o_i_ ?

 Complete this 9 letter word _a_s_o_i_ with the given clue.

1) girls love it
2) boys use it
3) parents hate it
4) mobiles scared of it

Meena’s father is Arun Puzzle

Meena's father is Arun.Then Arun is the ________of Meena's father.

1111=5, 2222=24, 3333=93, 4444=272, 5555=?

If 1111=5,

2222=24,

3333=93,

4444=272 then 5555=?